N 1 S 2 Sigma 2 Chi Squared

B X has a Nµσ2n distribution. 121 - One Variance.

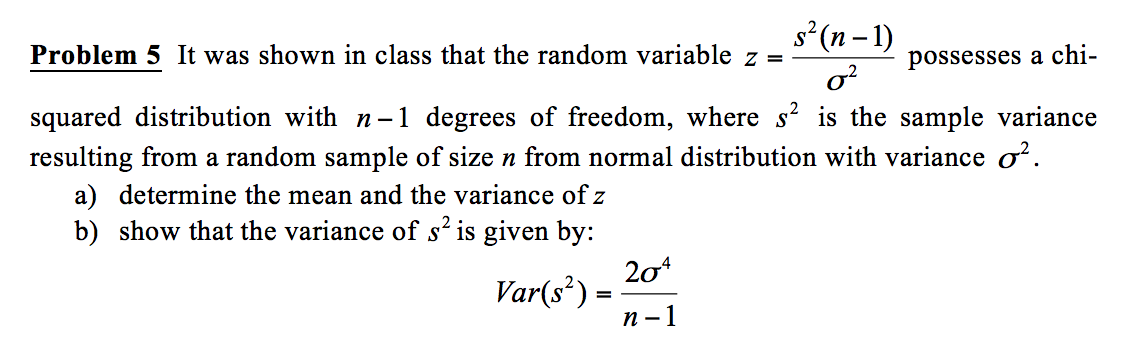
Solved It Was Shown In Class That The Random Variable Z Chegg Com

Then the sampling distribution of the sample variance is given by a chi-squared distribution with n1 n 1 degrees of freedom.

. Statistics and Probability questions and answers. So the numerator in the first term of W can be written as a function of the sample variance. It was shown in class that the random variable z s2 n - 1sigma2 possesses a chi-squared distribution with n - 1 degrees of freedom where s2 is the sample variance 2 resulting from a random sample of size n from normal distribution with variance sigma2.

If Xi χ21 and the Xi are independent then ni 1Xi χ2n. Step 3 Level of Significance. Make stats easy today with 1 stats help.

Because S is greater than σ this is a right tail test so df 11-110. Sigma 2 textright-tailed Step 2 Test Statistic. N 1S2s2 then the mgf of the sum on the left hand side is 1 2t 12ft Since the right hand side of the previous expression has mgf 1 2t n2 we must have ft 1 2t n21 2t 12 1 2t n 12 t.

The theoretical work for developing a hypothesis test for a population variance σ 2 is already behind us. There is a standard proof in elementary inferential statistics that proves that the estimator n - 1S 2 sigma 2 is chi-squared distributed with n - 1 degrees of freedom. Ad Dont fall behind in statistics.

Wide range of products. However this proof uses moment generating functions. Suppose that y 1 y n is a random sample from an N μ σ 2 distribution.

I 1 n y i y 2 σ 2. For all your research needs. T N-1ssigma_02 where N is the sample size and s is the sample standard deviation.

Available from Sigma-Aldrich to meet your research needs. The hypothesis testing problem is H_0. σ 12 7 2.

C n1S2σ2 has a chi-squared distribution with n1 degrees of freedom. Therefore if were interested in. W i 1 n X i μ σ 2 n 1 S 2 σ 2 n X μ 2 σ 2.

It assumes you already know the following. Ad Available from Sigma-Aldrich. Can anyone prove it for me.

The test statistic for testing above hypothesis testing problem is chi2 fracn-1s2sigma2 The test statistic follows a chi square distribution with n-1 degrees of freedom. Why is this the sum of χ 2 distributions that sum to a chi-square distribution with n 1 degrees of freedom instead n degree. The test statistic is.

Varmu u_i - mu Varu_i sigma2. N 1 S 2 i 1 n X i X 2. χ 2 n 1 S 2 σ 2 chi2frac n-1S2 sigma2 χ 2 σ 2 n 1 S 2.

The formula for a Chi-Square statistic is chi2 fracn-1s2sigma2. S 2 1 n 1 i 1 n X i X 2. The chi-squared distribution is a special case of the gamma distribution and is one of the most widely used probability distributions in.

V n 1 s2 σ2 χ2n 1. Show activity on this post. Frac n-1S2sigma2 sum_i1nleftfrac X_i-bar Xsigmaright2 equiv W_n sim chi2_n-1 with EW_n n-1.

Lets look at the chi square table. About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features Press Copyright Contact us Creators. The more this ratio deviates from 1 the more likely we are to reject the null hypothesis.

Varmu u_i - mu - 1n sum_jn u_j Var u_i 1 - 1n - 1 n sum_jin u_j sigma2 n-12 n2 n-1 n2 sigma2 n-1 n. If Z N0 1 then Z2 χ21. X 1n Pn i1 Xi and S 2 1n1 Pn i1Xi X2.

SDW_n sqrt 2n-1 Since this is a finite-sample result we can manipulate the exrpession without reservations. Parts a and c are proved as follows. ˉX the sample mean and S2 are independent.

4 4 V n 1 s 2 σ 2 χ 2 n 1. Has a χ n 1 2 distribution. In particular Q is a pivotal quantity since it is a function of the X_is and sigma2 and its distribution does.

Test statics is less than the critical value and it is not in rejection region. By Theorem 83 the random variable Q defined as beginequation Qfracn-1S2sigma2frac1sigma2sum_i1n X_i-overlineX2 endequation has a chi-squared distribution with n-1 degrees of freedom ie Q sim chi2n-1. And multiply both sides by n 1 we get.

Sigma 2 against H_1. If S 2 S2 S 2 is the variance of a random sample of size n n n taken from a normal population having the variance σ 2 sigma2 σ 2 then the statistic. A standard proof goes something like this.

The key element of this formula is the ratio sσ 0 which compares the ratio of the sample standard deviation to the target standard deviation. Recall that if you have a random sample of size n from a normal population with unknown mean μ and variance σ 2 then. S2 1 n1 n i1Xi X2.

The critical value for 95 confidence is 18307. S2 1 n1 Xn i1 Xi X2 1 n1. Then a X and S2 are independent random variables.

A χ2n random variable has the moment generating function 1 2t n 2. Lets take a look at the variance of y_i - mu. χ 2 n 1 S 2 σ 2.

Follows a chi-square distribution with n 1 degrees of freedom. In probability theory and statistics the chi-squared distribution also chi-square or χ 2-distribution with k degrees of freedom is the distribution of a sum of the squares of k independent standard normal random variables. Without loss of generality we assume that µ 0 and σ 1.

So for a single observation its smaller as expected and by the factor n-1 n. And the unbiased sample variance. Determine the mean and the variance of z.

The alternative hypothesis is H 1. Ok now what about y_i - ybar. 3 3 s 2 1 n 1 i 1 n X i X 2.

The independence of X and S2 can be established in other ways.

Solved Proof For Distribution Of N 1 S 2 Sigma 2 Is The Chi Chegg Com

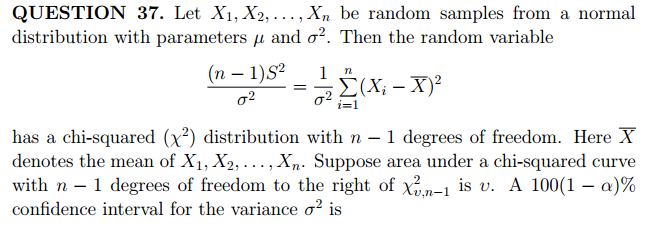
Solved Let X 1 X 2 X N Be Random Samples From A Chegg Com

Statistics Variance Of Sample Variance Mathematics Stack Exchange